Gradient - It's all downhill from now

The gradient operation acts on a scalar field \( f(x,y,z) \) producing a vector field \( \mathrm{grad}f(x,y,z) \). This vector field \( \mathrm{grad}f(x,y,z) \) at each location points in the direction of greatest increase of the scalar field at that location. The size of \( \mathrm{grad}f(x,y,z) \) is equal to the gradient in the direction of greatest increase. We shall prove this on the next page.

Consider the scalar field \(f(x,y,z)\), the gradient is given by operating del in the following way $$ \mathrm{grad} f(x,y,z) = \vec{\nabla}f(x,y,z) $$ $$= \Bigg(\frac{\partial }{\partial x} \hat{i} + \frac{\partial }{\partial y} \hat{j} + \frac{\partial }{\partial z} \hat{k} \Bigg) f(x,y,z) = \frac{\partial f(x,y,z)}{\partial x} \hat{i} + \frac{\partial f(x,y,z)}{\partial y} \hat{j} + \frac{\partial f(x,y,z)}{\partial z} \hat{k} $$

Consider a mountain range with the scalar field \( h(x,y) \) giving the height of the mountain range at every location \( (x,y) \), as illustrated below. The \( \mathrm{grad}h(x,y) \) produces a vector field that points directly up hill, perpendicular to contour lines (lines of equal height).

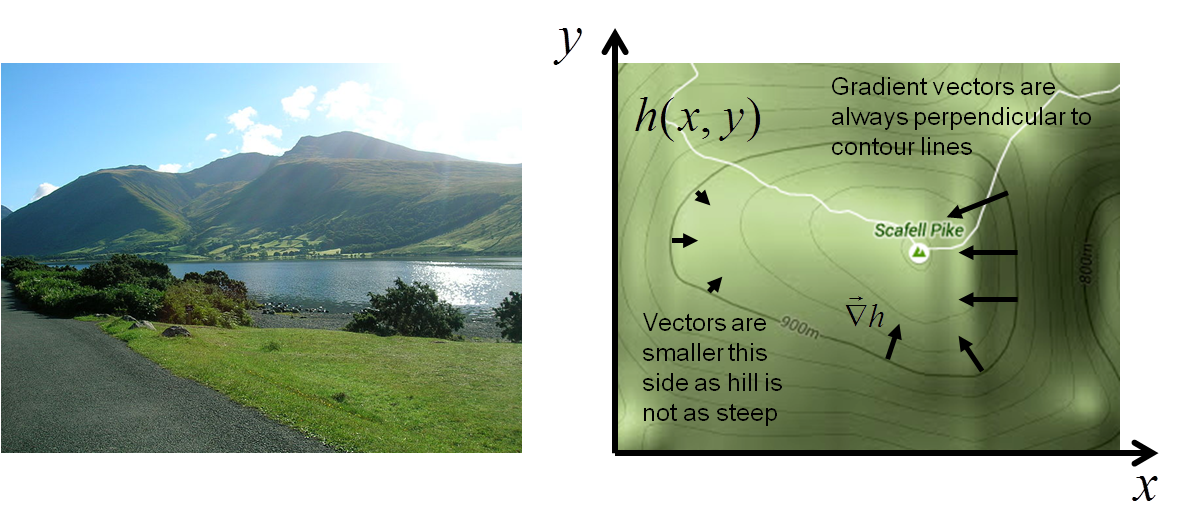
2D fields such as a hill can be represented with a contour plot.