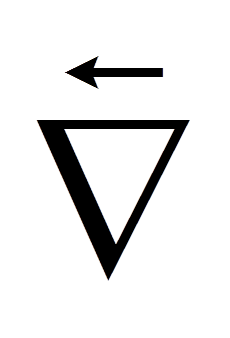Solutions

1. \( \frac{\partial \phi}{\partial x} = 2xy \), \( \frac{\partial \phi}{\partial y} = x^2 + z \) and \( \frac{\partial \phi}{\partial z} = y + 3z^2 \) so,

   \( \vec{\nabla}\phi = 2xy \hat{i} + (x^2+z)\hat{j} + (y+3z^2)\hat{k}.\)

2. \( \frac{\partial V}{\partial x} = x(x^2+y^2+z^2)^{-3/2} \), \( \frac{\partial V}{\partial y} = y(x^2+y^2+z^2)^{-3/2} \) and \( \frac{\partial V}{\partial z} = z(x^2+y^2+z^2)^{-3/2} \) so,

   \( \vec{E} = -\vec{\nabla}V = - \frac{x\hat{i} + y\hat{j} + z\hat{k}}{(x^2+y^2 + z^2)^{3/2}} \)

3. \( \frac{\partial z}{\partial x} = -2xh e^{-(x^2+2y^2)} \) and \( \frac{\partial z}{\partial y} = -4yh e^{-(x^2+2y^2)} \) so,

   \( \vec{\nabla} z = -2he^{-(x^2+2y^2)}(x\hat{i} + 2y\hat{j}) \).

   The gradient vectors point in the direction of greatest slope, therefore the lava must flow in the opposite direction to these vectors, hence parallel to \( (x\hat{i} + 2y\hat{j}) \).